How height is defined may not matter to you if you are tracking your running on a smart phone. However, if you are worried about how sea level rise may flood, say, the Netherlands, it matters a great deal. The key question is, height relative to what? In other words, what is your datum? In the context of a nautical chart, for example, the elevation of a light on a fixed structure is its height above mean high water (MHW), while the elevation of a light on a large navigational buoy is its height above mean sea level (MSL). The height of tree canopies is typically referenced to height above local ground elevation, and pilots distinguish between an aircraft’s above ground level (AGL) altitude and its MSL altitude.
MSL can be defined as the arithmetic mean of hourly heights of the sea at a primary-control tide station observed over a period of 19 years—which is how long it takes the moon to go through all the possible combinations of its cycles with respect to the sun and Earth. However, MSL is not the same everywhere, because the topography of the sea’s surface is affected by local temperature, density, salinity, currents, wind, and other physical forces. Additionally, carrying that datum inland, to the middle of a continent, is extremely difficult.
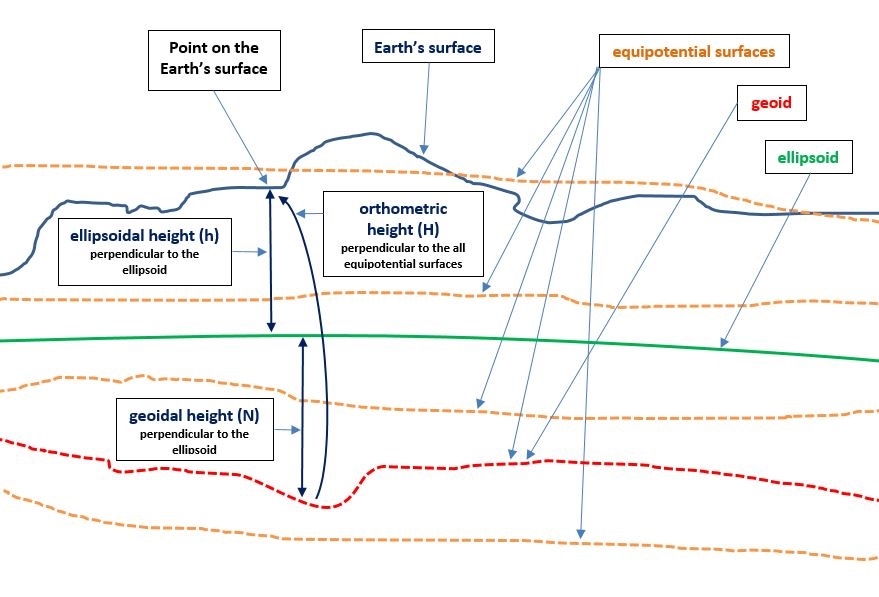
Therefore, in today’s world of surveying, GIS, and GNSS, we speak of three other types of height: ellipsoidal height (h; aka geodetic height), geoidal height (N; aka geoid undulation), and orthometric height (H). These three heights are related by the equation H = h – N.
Ellipsoidal Height
GNSS receivers measure height from a reference ellipsoid—or flattened sphere—which is a geometric model of Earth. The ellipsoidal height is the distance between a point on this ellipsoid, as represented by a coordinate pair, and a corresponding point on Earth’s surface, as measured along a line perpendicular to the ellipsoid. If at a given place the reference ellipsoid is below Earth’s surface, the ellipsoidal height has a positive sign; if it is above Earth’s surface, the ellipsoidal height has a negative sign.
Ellipsoidal heights are not affected by any physical forces, because the ellipsoid is a smooth mathematical construct with a shape based on a hypothetical equipotential gravitational surface. In other words, it assumes that Earth’s gravity field is smooth and continuous.
Because measurement is along a line perpendicular to the ellipsoid, it is impossible to measure the direction of this line with an instrument. Yet, ellipsoidal heights can be readily calculated by GPS receivers (conversely, they could not be easily determined prior to the advent of GPS in the 1980s).
Around the world there are many horizontal datums, each utilizing a specific reference ellipsoid. These reference ellipsoids have slightly different shapes and/or origins. Therefore, the term ellipsoidal height is ambiguous unless one also specifies which horizontal datum one is using. For example, GPS uses as its horizontal datum the World Geodetic System of 1984 (WGS84). In turn, GPS data has helped to improve WGS84, including bringing the ellipsoid’s center closer to Earth’s actual center of mass. This geocenter is the origin of all satellites’ orbits, of the measurements derived from them, and of 3D Cartesian coordinates—X, Y, and Z—often used to express GPS observations.
Manufacturers of GPS receivers load them with a crude global geoid model, which they use to convert the GPS-derived WGS84 ellipsoidal height into a (low accuracy) elevation.
Geoidal Height
The geoid is a representation of what the sea surface around the planet would be—rising and falling with local variations in gravity—if it were not for tides and currents. Using complex math and gravity readings on land, surveyors extend this imaginary line through the continents. The geoid, which reflects Earth’s irregular shape and distribution of mass, is the continuously evolving product of research in physical geodesy, aided greatly in recent years by gravimetric stations and satellite altimetry missions.
A geoidal height is the distance, measured along a line perpendicular to the ellipsoid of reference, from the ellipsoid to the geoid. It is a positive or negative number, depending on whether the ellipsoid at any given location is above or below the geoid. Throughout the coterminous United States, the geoid is below the ellipsoid.
To produce accurate elevations, surveyors set the appropriate geoid model within their RTK collection software or static post-processing software.
Orthometric Height
Orthometric heights—more commonly called elevations—are measured along a plumb line from the geoid to a point on Earth’s surface.
Shift to Geoid-Based Vertical Datum
Both Canada and the United States have been shifting to a geoid-based vertical datum. In the spring of 2012, the two countries agreed to define, realize, and maintain a common vertical datum by 2022 based on a common geoid model and to define this vertical datum by the equipotential surface 62,636,856.00 m2s-2 representing the coastal MSL for North America along the Pacific, Atlantic, and Gulf coasts.